stillvue.blogg.se - Binomial cdf formula

Specifically, when np > 5 or n(1-p)>5 the Normal Approximation method should not be used. When p is very small or very large, the Normal Approximation starts to suffer from increased inaccuracy. However, there are times when the Normal Distribution is not a good estimator of the Binomial. While the use of the Normal Distribution seems odd at first, it is supported by the central limit theorem and with sufficiently large n, the Normal Distribution is a good estimate of the Binomial Distribution.

The use of the z value from the Normal Distribution is where the method earns its moniker “Normal Approximation”. While this method is very easy to teach and understand, you may have noticed that z1- α/2 is derived from the Normal Distribution and not the Binomial Distribution. Thus, we would be 95% confident that the proportion of the target population (all voters in California) who intend to vote for Mr. Gubinator, the resulting 95% CI would be calculated as follows. Using our previous example, if a poll of 50 likely voters resulted in 29 expressing their desire to vote for Mr.

z 1- α/2 = “z value” for desired level of confidence.

The equation for the Normal Approximation for the Binomial CI is shown below. Normal Approximation Method of the Binomial Confidence Interval There are several ways to estimate the Binomial Confidence Interval (CI) in this article we will focus on the Normal Approximation Method and the Clopper-Pearson Method. The margin of error is also called the confidence interval and is used to describe how much uncertainty we have in the sample estimate. This +/-5% indicates that if the poll was repeated multiple times, the result would likely fall in the range of 58% +/- 5%, or 53% to 63%. When polls are presented in the media, on the bottom of the screen or page you often see a small note with wording similar to “Margin of error +/- 5%”. The poll and most binomial samples come with some error. If we were to repeat this poll several times in the same day (using a different group of 50 each time) we would find that the percentage that intends to vote for Mr. Does this mean that 58% of all voters intend to vote for Mr.

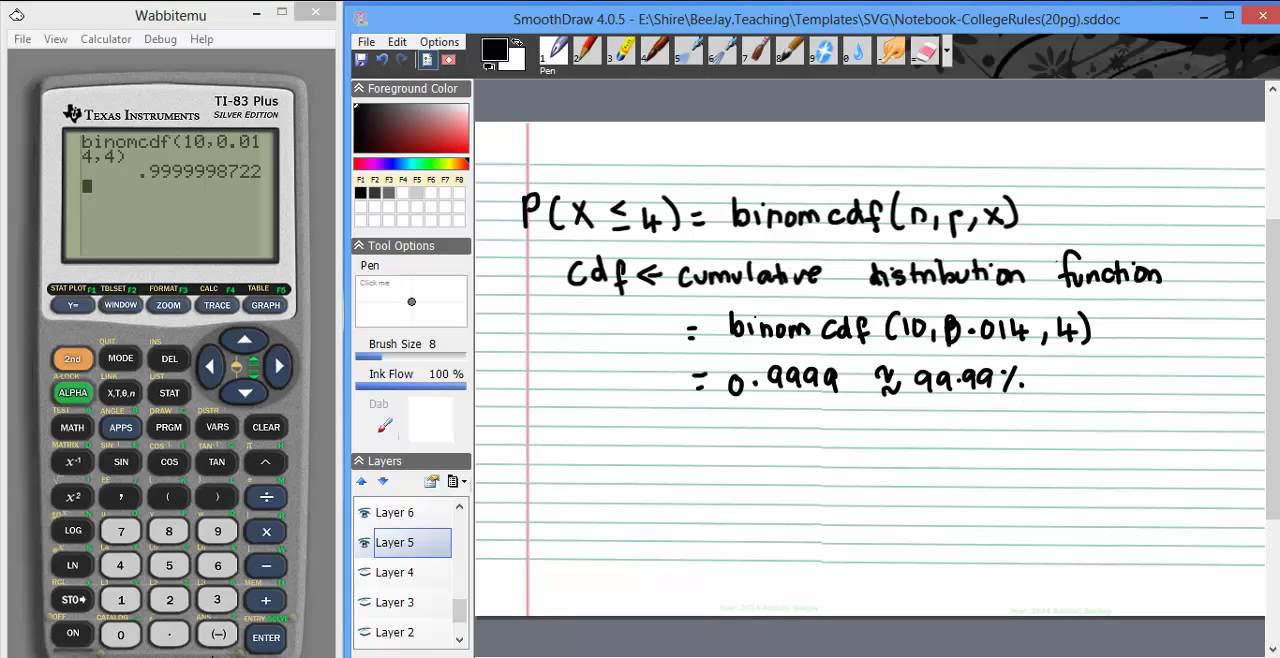
In our poll of 50 likely voters, 58% indicate they intend to vote for Mr. If the poll gives the voters a choice between the two candidates, then the results can be reasonably modeled with the Binomial Distribution. We would like to know who is winning the race, and therefore we conduct a poll of likely voters in California. To illustrate this, let’s assume that two candidates are running in an election for Governor of California. Polling organizations often take samples of “likely voters” in an attempt to predict who will be elected before the actual election occurs. For example, in the election of political officials we may be asked to choose between two candidates. Binomial data and statistics are presented to us daily. The Binomial Distribution is commonly used in statistics in a variety of applications. Healthcare, Medical Devices, and Pharmaceutical Statistics Training.